A fractal can easily be described as a self-repeating similar geometric pattern. I’m no expert by any means, but I have always been interested in these less traditional topics in mathematics. The first fractal that I remember coming across was the Koch snowflake, one of the earliest fractal patterns to be described. In this fractal, an equilateral triangle is taken and altered in such a way to create an infinite self-repeating fractal pattern. Another well-known fractal is the Sierpinski triangle. This is also an equilateral triangle, recursively subdivided into infinitely smaller equilateral triangles. There is also a three dimensional Sierpinski square pyramid, with fractal patterns similar to the two dimensional triangle. This illustrates the fact that fractals can be taken into higher dimensions, like the background to Gabi Asfour’s Fall 2014 Topo-Graphic fashion film. I noticed the fractal patterns when I first saw the film being screened. This fashion film has become one of my favorite non-narrative fashion films I have seen so far because of its innovative use of geometric fractal patterns.
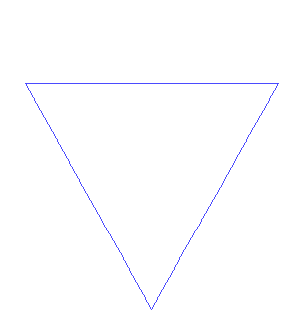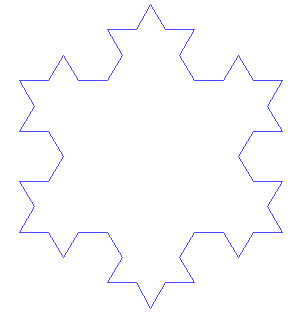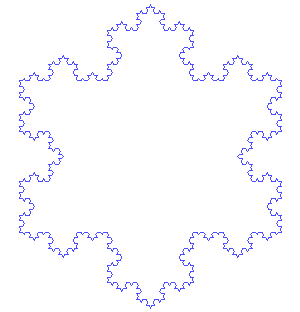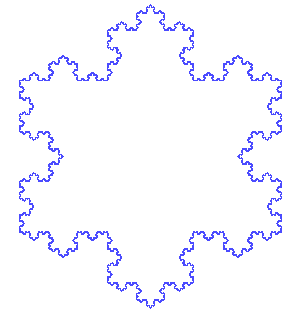
The first 7 iterations of the Koch Snowflake.
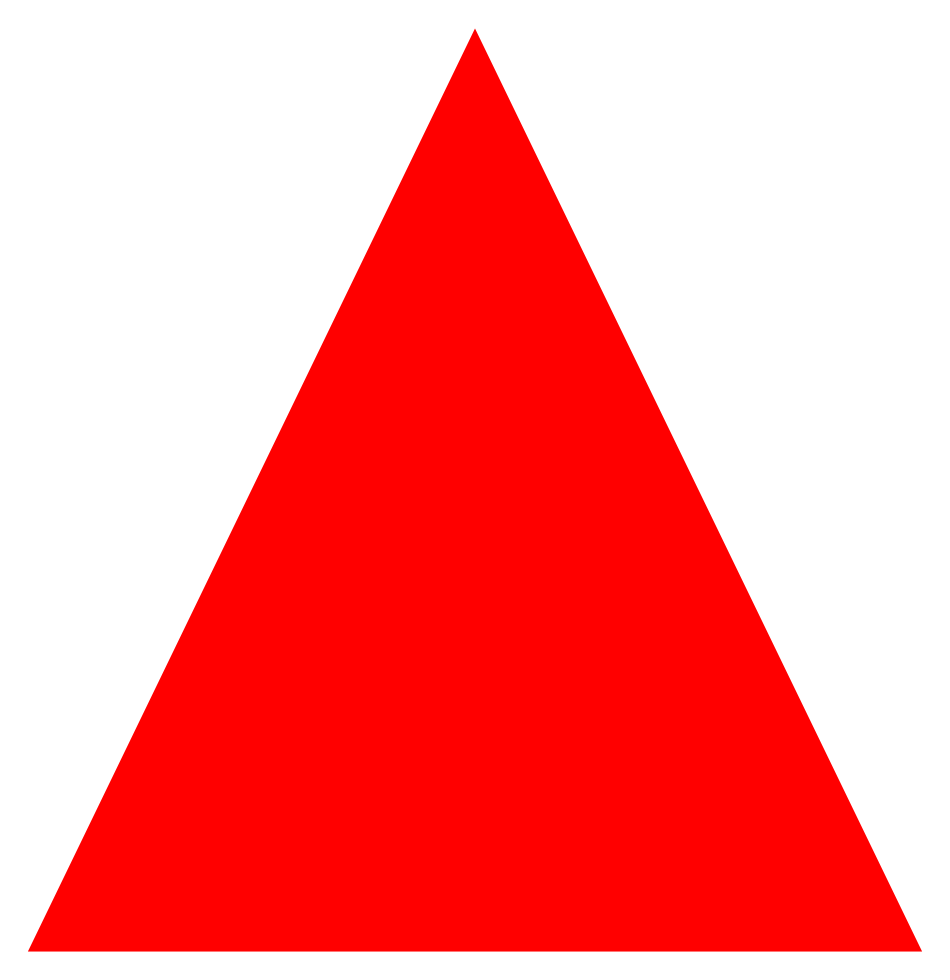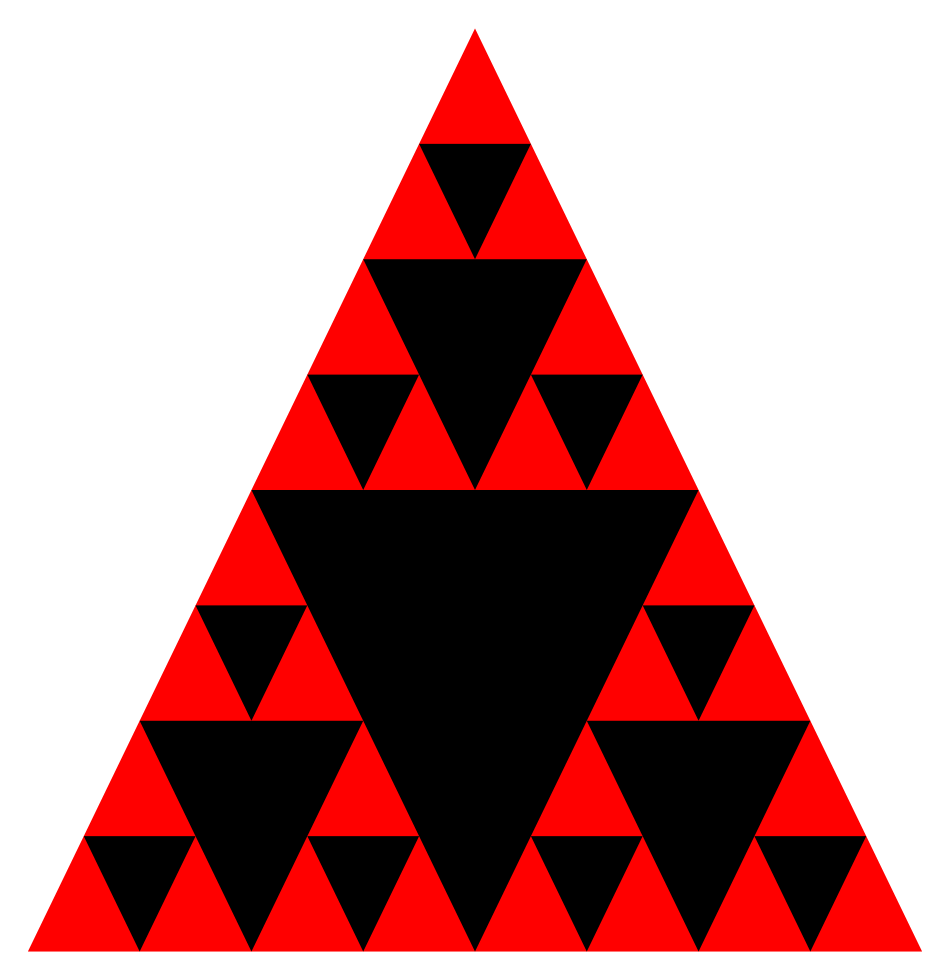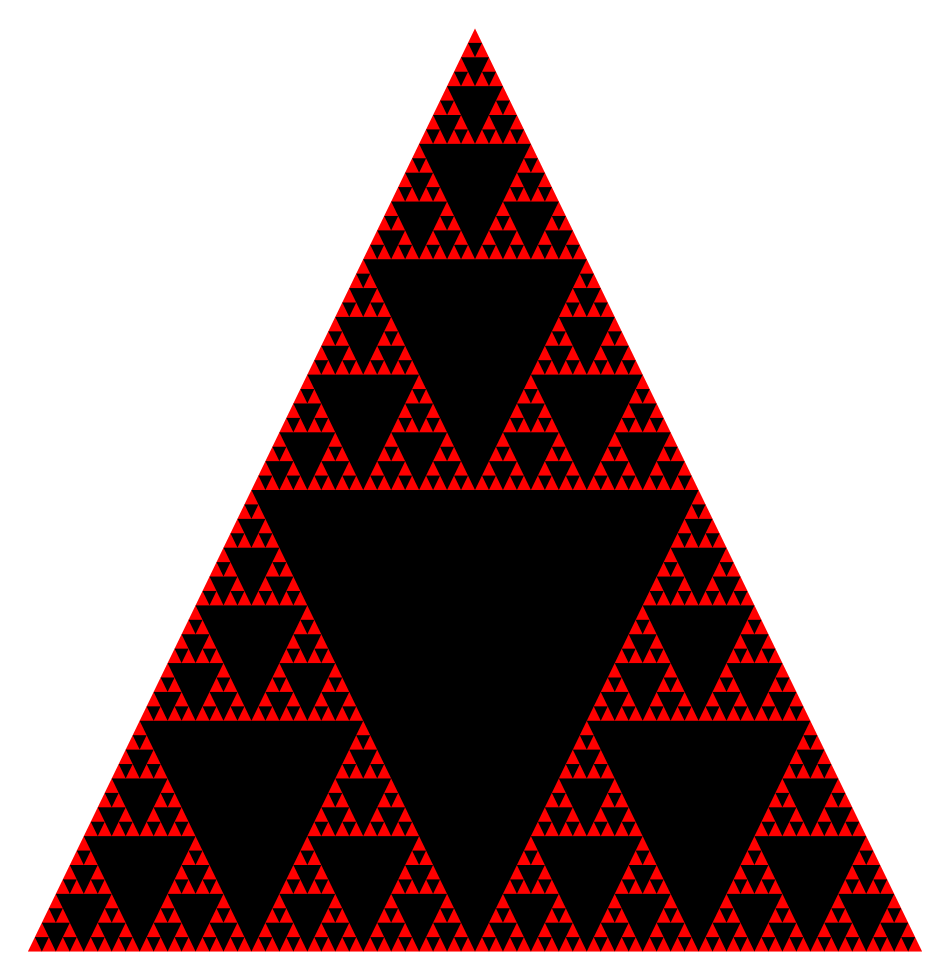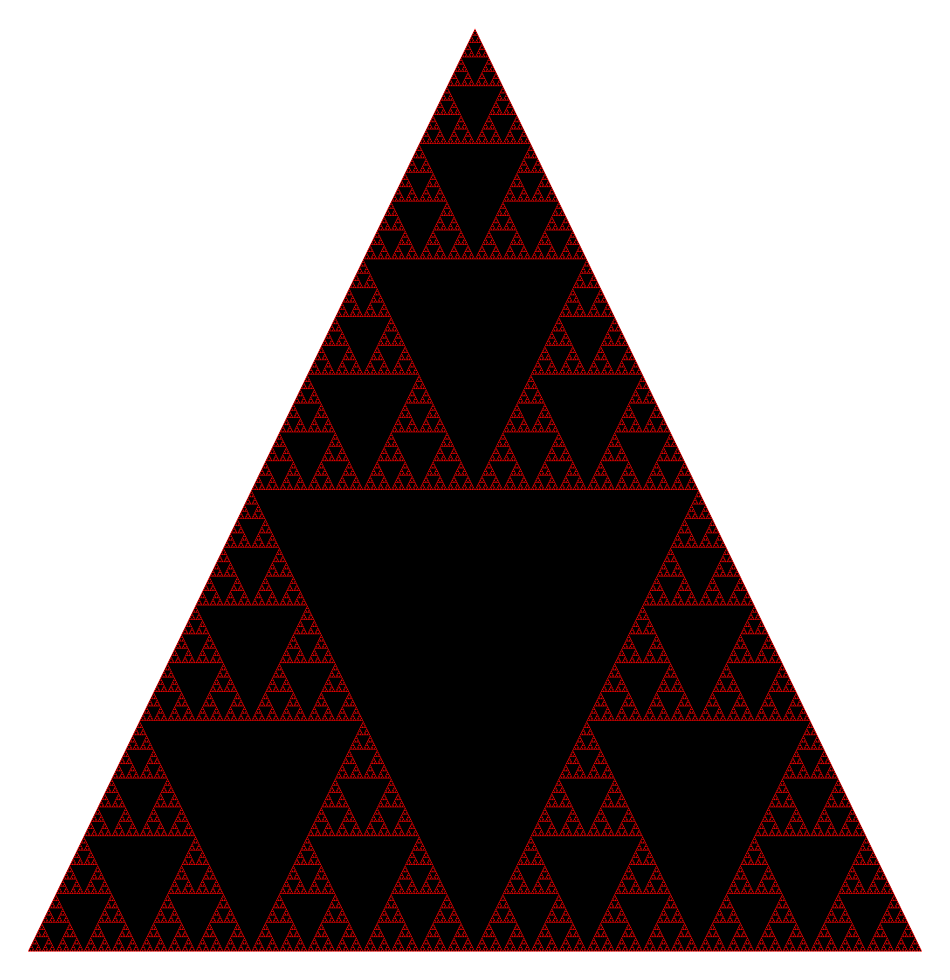
Sierpinski Triangle

Sierpinski Square Pyramid
Click here to see the threeASFOUR Topo-Graphic Fashion Film
