“Huh?”
Yeah, that’s what I thought too. It’s possibly the single least likely phrase I expected to stumble upon in a book about an artist. But there it was. Out of context, I suppose it really doesn’t make any sense. But if I said that the book I was reading was about M.C. Escher (called The Magic of M.C. Escher), it would probably be an “Aha!” moment.
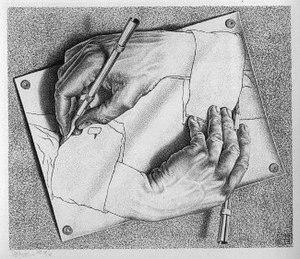
Escher's famous "Drawing Hands" explores the idea of paradoxes and "strange loops"
The well-known, innovative 20th century artist M.C. Escher was the creator of, in a phrase, “art for science and math lovers.” Infinity, polyhedra, geometric distortions, tessellations, impossible constructions – they’re all there somewhere in his work. Crazy, no? He didn’t even have any formal mathematical training. That’s even crazier.
I cannot pretend to comprehend much of what I read about him. There isn’t exactly a lot of discussion about technique and aesthetic. Instead, it’s more about Penrose triangles and orthogonal forces of gravity. Not quite the type of thing that I like to involve myself with. I have nothing against it. I just admittedly don’t understand much of it. That’s what I think makes Escher so remarkable. I’ve spoken to quite a few Mathletes about art, and many of them told me that they just don’t “get” it. It’s markedly outside their comfort zone. Well, here comes Escher to the rescue. Scientists and mathematicians arguably enjoy his work more than anybody else.
It’s a remarkable feat, but Escher effectively managed to tether the worlds of art and technical logistics in a way that I do not believe anyone ever has. I mean, it’s art that penetrates a world that is inhabited by its theoretical opposite. In that way, it’s art that almost transcends the barriers that usually serve to confine it. We’ve talked a lot about the ever-expanding artistic intersection in our class – which includes politics, industry, and commerce, among others – and I think that Escher, with his mind-boggling work, adds mathematics to the list. So to those with a penchant for science I say you’ve found a remarkable associate in Escher. To those who are not quite so scientifically inclined (myself included) I say Escher is yet another brilliant artist to marvel at.


The beauty of Escher is not in understanding his methods and mathematical genius. It is precisely in not understanding. The confusing and often puzzling works of Escher highlight our ability, or inability, to perceive depth at a 2 dimensional level is in itself fantastic and bewildering. The way that he uses this in conjunction with his brilliantly put together pieces makes his work what it is. The technique is only as effective as its affect, and Escher has successfully used a great technique to fantastic affect.
Math truly runs the art world. As musicians and composers know, it rules of mathematics apply when creating and timing pieces. Escher’s “Drawing Hands” clearly shows a mastery of using different perspectives and shading to create a work of art that literally stands out. In many of M.C. Escher’s works, he effectively displays mastery of intesselations, shaping of space and polyhedrons. Often when examining art, I am truly fond of the way artists manipulate geometry, including ratios. Another artist I know does this well is Salvador Dali. For example, in his famous “The Sacrament of the Last Supper, ”Christ and his disciples are pictured inside a giant dodecahedron.
The way math and science and even psychology interact with art is fascinating. In my psychology class in high school, we learned about how the brain uses rules of perception to make sense of the images that our eyes take in. In order to better understand the concept, we studied paintings and drawings in which the artist intentionally manipulated the perception rules. The results were things like staircases that have no end and waterfalls flowing uphill. It is all fascinating stuff, the way that art can mess with your brain and perceive things that don’t make any sense and could not actually exist in the physical world.
It may be hard to understand, but to simplify the idea of Escher’s art, it is important to understand the title of this blog post. A Dodecahedron is a three-dimensional shape consisting of twelve regular pentagonal faces, and stellation is the construction of the dodecahedrons in a way that extends the elements, such as edges or planes, in a symmetrical way until they meet each other again. This idea explains “Drawing Hands.”
When you understand this concept, it is much easier to appreciated Escher’s work. My favorite piece, though, is “Relativity,” because it is so much more complex and even mind-boggling to imagine how he calculated the shapes and patterns of his drawing. I could look at it forever, in fact, and never get bored. Here is a link to “Revativity” so you can see what I mean: http://blog.art21.org/2010/08/03/the-paradoxical-art-of-inception/relativity-escher/.
This certainly is interesting. In a society where abstract art, which tends to forgo perspective and such, runs rampant, this more complicated, complex artwork is refreshing. Refreshing? Yes, simply because it is different from most of what we have seen. This piece is also intriguing because, as Ana blogged, the process of creating a piece of art is extremely important in the final product. Although I have seen this drawing countless times in school, I never knew the complexity behind its creation. I never really knew anything about this artist, actually, and I love that Rosie shared the link to another of Escher’s pieces. This concept is similar to the performance from the Australian Ballet that we saw as a class at Fall for Dance. At a more superficial level, the dance looked beautiful; it was nice. However, as we learned, ballet has five central footing positions, in addition to countless other technical aspects. For something that initially seemed like “nice shading,” there is an ample amount of additional respect that I now have for this piece, knowing the technicality behind it.